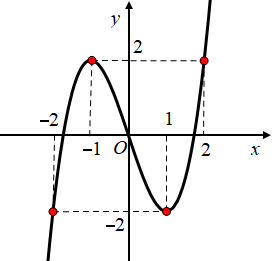
Cho đa thức bậc ba $\large y = f(x)$ có đồ thị hàm số như hình vẽ sau

Cho đa thức bậc ba $\large y = f(x)$ có đồ thị hàm số như hình vẽ sau

Có bao nhiêu giá trị nguyên không âm của tham số $\large m$ để phương trình $\large f \left(\sqrt {f(sin 2x) + 2}\right) = f\left(\dfrac {m}{2}\right)$ có nghiệm thuộc nửa khoảng $\large \left(- \dfrac {\pi}{4}; \dfrac {\pi}{4}\right]$

• A.

  3

• B.

  4

• C.

  2

• D.

  1

Ta có $\large \left(- \dfrac {\pi}{4}; \dfrac {\pi}{4}\right] \Rightarrow -1 < sin 2x \leq 1 \Rightarrow  -2 \leq f(sin 2x) < 2 \Rightarrow 0 \leq f(sin 2x) + 2 < 4$
$\large \Rightarrow 0 \leq \sqrt {f(sin 2x) + 2}  < 2 \Rightarrow -2 \leq f \left(\sqrt {f(sin 2x) + 2}\right) < 2$
Để phương trình $\large f \left(\sqrt {f(sin 2x) + 2}\right) = f\left(\dfrac {m}{2}\right)$ có nghiệm thì $\large -2 \leq f\left(\dfrac {m}{2}\right) < 2$
Tức là $\large -2 \leq f\left(\dfrac {m}{2}\right) < 2 \left\{\begin{matrix}  -2 \leq \dfrac {m}{2} < 2 \\ \dfrac {m}{2} \neq -1 \end{matrix}\right. \Rightarrow \left\{\begin{matrix}  -4 \leq m < 4 \\ m \neq  -1 \end{matrix}\right.$
Mà $\large m$ không nguyên âm vậy $\large m \in \{0;1;2;3\}$. Vậy có 4 giá trị