Cho khối chóp S.ABCD có đáy hình chữ nhật, $\large AB=a,AD=a\
Lưu về Facebook:

MỤC LỤC
Câu hỏi:
Cho khối chóp S.ABCD có đáy hình chữ nhật, AB=a,AD=a√3,SA vuông góc với đáy và mặt phẳng (SBC) tạo với đáy một góc 60∘. Tính thể tích V của khối chóp S.ABCD.
Đáp án án đúng là: C
Lời giải chi tiết:
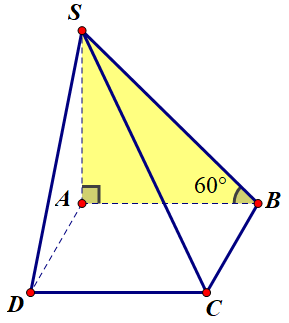
Do BC⊥(SAB)⇒((SBC),(ABCD))=^SBA=60∘
Ta có: SA=AB.tan^SBA=a√3
SABCD=AB.AD=a2√3.
Suy ra: V=13SA.SABCD=13⋅a√3⋅a2√3=a3
Đáp án C
Xem thêm các bài tiếp theo bên dưới